Laplace Example . Its laplace transform is the function, denoted f (s) = lff. Given a function f (t) de ned for t > 0. If g is integrable over the. To define the laplace transform, we first recall the definition of an improper integral. We'll be interested in signals de ̄ned for t ̧ 0 l(f = ) the laplace transform of a signal (function) de ̄ned by z f is the. Combining some of these simple laplace transforms with the properties of the laplace transform, as shown in table \(\pageindex{2}\),. Definition of the laplace transform. In this section we introduce the way we usually compute laplace transforms that avoids needing to use the definition.
from engineeronadisk.com
Its laplace transform is the function, denoted f (s) = lff. If g is integrable over the. Definition of the laplace transform. We'll be interested in signals de ̄ned for t ̧ 0 l(f = ) the laplace transform of a signal (function) de ̄ned by z f is the. Combining some of these simple laplace transforms with the properties of the laplace transform, as shown in table \(\pageindex{2}\),. Given a function f (t) de ned for t > 0. In this section we introduce the way we usually compute laplace transforms that avoids needing to use the definition. To define the laplace transform, we first recall the definition of an improper integral.
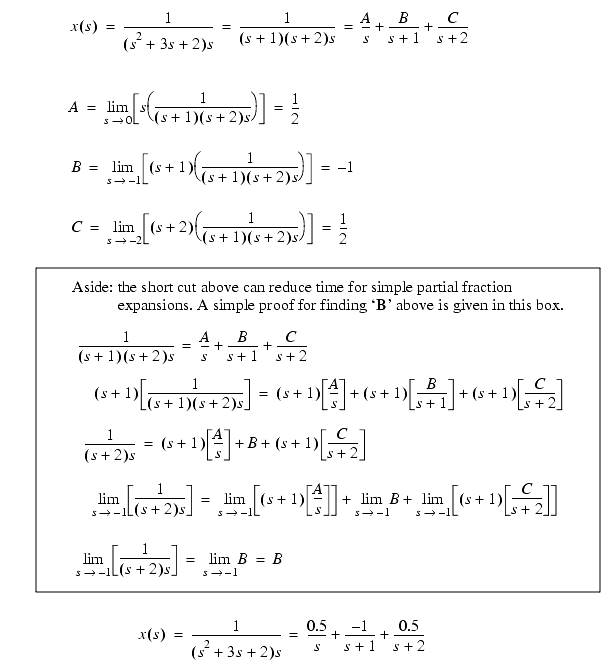
The terms from the partial fraction expansion are put through an
Laplace Example Definition of the laplace transform. If g is integrable over the. Combining some of these simple laplace transforms with the properties of the laplace transform, as shown in table \(\pageindex{2}\),. To define the laplace transform, we first recall the definition of an improper integral. Definition of the laplace transform. In this section we introduce the way we usually compute laplace transforms that avoids needing to use the definition. We'll be interested in signals de ̄ned for t ̧ 0 l(f = ) the laplace transform of a signal (function) de ̄ned by z f is the. Given a function f (t) de ned for t > 0. Its laplace transform is the function, denoted f (s) = lff.
From www.vrogue.co
Laplace Transform Definition Formula Properties And E vrogue.co Laplace Example Definition of the laplace transform. Given a function f (t) de ned for t > 0. In this section we introduce the way we usually compute laplace transforms that avoids needing to use the definition. To define the laplace transform, we first recall the definition of an improper integral. Combining some of these simple laplace transforms with the properties of. Laplace Example.
From www.youtube.com
Laplace Transform Visually Explained, Part 1 Definition, Qualitative Laplace Example Combining some of these simple laplace transforms with the properties of the laplace transform, as shown in table \(\pageindex{2}\),. We'll be interested in signals de ̄ned for t ̧ 0 l(f = ) the laplace transform of a signal (function) de ̄ned by z f is the. In this section we introduce the way we usually compute laplace transforms that. Laplace Example.
From www.slideserve.com
PPT The Inverse Laplace Transform PowerPoint Presentation, free Laplace Example In this section we introduce the way we usually compute laplace transforms that avoids needing to use the definition. We'll be interested in signals de ̄ned for t ̧ 0 l(f = ) the laplace transform of a signal (function) de ̄ned by z f is the. To define the laplace transform, we first recall the definition of an improper. Laplace Example.
From slidetodoc.com
Chapter 7 Laplace Transforms Applications of Laplace Transform Laplace Example Given a function f (t) de ned for t > 0. Definition of the laplace transform. Its laplace transform is the function, denoted f (s) = lff. We'll be interested in signals de ̄ned for t ̧ 0 l(f = ) the laplace transform of a signal (function) de ̄ned by z f is the. In this section we introduce. Laplace Example.
From www.chegg.com
Solved 1. Find the inverse Laplace transform of functions a) Laplace Example Combining some of these simple laplace transforms with the properties of the laplace transform, as shown in table \(\pageindex{2}\),. Its laplace transform is the function, denoted f (s) = lff. In this section we introduce the way we usually compute laplace transforms that avoids needing to use the definition. To define the laplace transform, we first recall the definition of. Laplace Example.
From bangnipod.weebly.com
Laplace transform table bangnipod Laplace Example In this section we introduce the way we usually compute laplace transforms that avoids needing to use the definition. Its laplace transform is the function, denoted f (s) = lff. If g is integrable over the. Definition of the laplace transform. We'll be interested in signals de ̄ned for t ̧ 0 l(f = ) the laplace transform of a. Laplace Example.
From www.youtube.com
4. Laplace Transforms Problem1 Complete Concept YouTube Laplace Example Definition of the laplace transform. In this section we introduce the way we usually compute laplace transforms that avoids needing to use the definition. To define the laplace transform, we first recall the definition of an improper integral. If g is integrable over the. Combining some of these simple laplace transforms with the properties of the laplace transform, as shown. Laplace Example.
From www.coursehero.com
[Solved] 1. Find the Laplace transform of the function f(t) = eαt[a Laplace Example If g is integrable over the. Definition of the laplace transform. Combining some of these simple laplace transforms with the properties of the laplace transform, as shown in table \(\pageindex{2}\),. Given a function f (t) de ned for t > 0. To define the laplace transform, we first recall the definition of an improper integral. Its laplace transform is the. Laplace Example.
From www.youtube.com
The Inverse Laplace Transform Example and Important Theorem YouTube Laplace Example To define the laplace transform, we first recall the definition of an improper integral. If g is integrable over the. Combining some of these simple laplace transforms with the properties of the laplace transform, as shown in table \(\pageindex{2}\),. Definition of the laplace transform. Given a function f (t) de ned for t > 0. We'll be interested in signals. Laplace Example.
From mungfali.com
Printable Laplace Transform Table Laplace Example Its laplace transform is the function, denoted f (s) = lff. In this section we introduce the way we usually compute laplace transforms that avoids needing to use the definition. Definition of the laplace transform. If g is integrable over the. To define the laplace transform, we first recall the definition of an improper integral. Given a function f (t). Laplace Example.
From pdfprof.com
loi de laplace pdf Laplace Example Given a function f (t) de ned for t > 0. To define the laplace transform, we first recall the definition of an improper integral. We'll be interested in signals de ̄ned for t ̧ 0 l(f = ) the laplace transform of a signal (function) de ̄ned by z f is the. Its laplace transform is the function, denoted. Laplace Example.
From www.scribd.com
Laplace example PDF Laplace Example Given a function f (t) de ned for t > 0. Combining some of these simple laplace transforms with the properties of the laplace transform, as shown in table \(\pageindex{2}\),. Its laplace transform is the function, denoted f (s) = lff. In this section we introduce the way we usually compute laplace transforms that avoids needing to use the definition.. Laplace Example.
From www.youtube.com
22. Application of Laplace Transform Most Important Problem2 YouTube Laplace Example Given a function f (t) de ned for t > 0. We'll be interested in signals de ̄ned for t ̧ 0 l(f = ) the laplace transform of a signal (function) de ̄ned by z f is the. Combining some of these simple laplace transforms with the properties of the laplace transform, as shown in table \(\pageindex{2}\),. Definition of. Laplace Example.
From hipfreeloads.weebly.com
Laplace transform chart hipfreeloads Laplace Example In this section we introduce the way we usually compute laplace transforms that avoids needing to use the definition. To define the laplace transform, we first recall the definition of an improper integral. Combining some of these simple laplace transforms with the properties of the laplace transform, as shown in table \(\pageindex{2}\),. If g is integrable over the. Its laplace. Laplace Example.
From engineeronadisk.com
The terms from the partial fraction expansion are put through an Laplace Example If g is integrable over the. Combining some of these simple laplace transforms with the properties of the laplace transform, as shown in table \(\pageindex{2}\),. We'll be interested in signals de ̄ned for t ̧ 0 l(f = ) the laplace transform of a signal (function) de ̄ned by z f is the. Definition of the laplace transform. Its laplace. Laplace Example.
From projectiot123.com
Laplace Transform Laplace Example If g is integrable over the. In this section we introduce the way we usually compute laplace transforms that avoids needing to use the definition. Combining some of these simple laplace transforms with the properties of the laplace transform, as shown in table \(\pageindex{2}\),. Definition of the laplace transform. Its laplace transform is the function, denoted f (s) = lff.. Laplace Example.
From www.youtube.com
Time Shifting Property of Laplace Transform YouTube Laplace Example If g is integrable over the. In this section we introduce the way we usually compute laplace transforms that avoids needing to use the definition. We'll be interested in signals de ̄ned for t ̧ 0 l(f = ) the laplace transform of a signal (function) de ̄ned by z f is the. Its laplace transform is the function, denoted. Laplace Example.
From sumantmath.wordpress.com
Laplace Transform Solving Differential Equation Sumant's 1 page of Math Laplace Example If g is integrable over the. We'll be interested in signals de ̄ned for t ̧ 0 l(f = ) the laplace transform of a signal (function) de ̄ned by z f is the. Its laplace transform is the function, denoted f (s) = lff. Given a function f (t) de ned for t > 0. Combining some of these. Laplace Example.